App 2.1: Development of equations for calculating pool sizes from isotope dilution
App 2.2: References
Contributor: Andy Coward
In much of the literature (see Chapter 4 & ref 1) the relationship for calculating total body water from the enrichment of 2H or 18O in a urine, saliva or breath sample takes the form:
![]()
.........1
where f is a fractionation factor for the biological material analysed and APEa is the atom % excess of the dose given. Rst is the absolute isotopic ratio in the standard against which the enrichment d (‰) of post-dose and pre-dose samples are measured. Converting to moles we obtain:
![]()
.........2
In contrast, for work with man, we recommend a relationship similar to that described by Halliday and Miller ² namely:
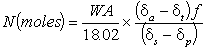
.........3
That these equations are equivalent or even appropriate may not be immediately obvious. Equation 2 is somewhat confusing because no less than three types of unit are used to describe isotopic content, namely APE, d and R. Furthermore, an investigator new to the field is given no indication of how APEa or MWta is to be calculated and the temptation must be to read these values off the label on the bottle of isotope. Such a manoeuvre is fraught with danger because it assumes that both the isotope manufacturer and the investigator can get the same value for an analysis. This may or may not be the case. For these reasons the procedure we describe insists that the isotope content of the dose given is measured and, for clarity all the units used for expressing isotopic content are the same.
The principle of any dilution procedure for measuring the size of a single pool is that the increase in the amount of isotope within the pool following dose administration is equal to the amount of isotope given. We need therefore, to measure each of these quantities.
Increase of isotope within the pool is equal to the peak isotopic enrichment found after the dose is given minus the amount that was there as background before the dose was given. The peak enrichment is the intercept determined from back-extrapolation of the isotope curve to time zero in the case of the slope-intercept method, and the isotope enrichment of the plateau measurement in the case of the 2-point technique.
If we assume that the amount of dose is small relative to pool size we can write:
NCs - NCp
or N(Cs - Cp)
.........4
for the amount of isotope gained, where C is fractional abundance (see Section 3.3.3).
To derive a value for the amount of isotope given the isotope concentration in the dose should also be measured. This can be conveniently done by diluting a small weight of dose in a large weight of water in the same way that body-water dilutes the dose given. Making the assumption that the amount of dose diluted (a, grams) is small relative to the amount of water used for the dilution (W, grams of molecular weight 18.02) we can therefore write:
![]()
.........5
for the amount of isotope derived from (a) of dose. Thus the amount of isotope given is:
![]()
.........6
The molecular weight terms cancel giving:
![]()
.........7
Putting Equation 4 equal to Equation 7 (since dose found equals dose given) and re-arranging we derive:
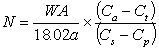
.........8
and if the sample analysed is fractionated relative to body water then:
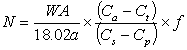
.........9
in which the term (Ca - Ct)/(Cs - Cp) can be expressed in terms of isotopic ratios (R). For 2H the general substitution is:
![]()
.....(see Section 3.3.3) .........10
and this gives:
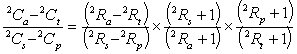
.........11
or:
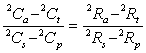
.........12
Substitution of typical values for Rs, Rp, Ra, Rt justifies this approximation.
Similarly, for 18O where:
![]()
.........14
it can be shown that:
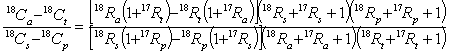
.........15
Again, substitution of typical values justifies:
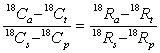
.........16
To convert isotope ratios to relative delta per mil values the following substitution can be applied (see Section 3.3.3):
![]()
.........17
In which case, for both 2H and 18O
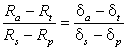
.........18
giving the equation for total body water described earlier as Equation 3:
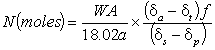
.........3
The reader, now familiar with the relationships between d, R and C will be able to understand the origin of both Equation 2 and Equation 3. Equation 3 is justified if the ranges of enrichments measured are sufficiently small to replace all measurements of change in isotope concentration with change in isotope ratio. A similar approximation exists in Equation 2 because the amount of isotope found in the subject after dose administration is calculated as:
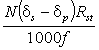
.........19
which is the same as N(Rs-Rp)/f rather than N(Cs - Cp)/f and this value is divided into the amount of dose given expressed in units of isotope concentration.
Users of the multi-point methodology
will find it convenient to use the reciprocal of Equation 3 for all values of isotopic
enrichment obtained during the measurement period. This measurement is equivalent to
isotopic concentration (mole/mole body water) expressed as fraction of the dose given and
the intercept of the log-linear plot of such values is 1/N (see Chapter 11 for examples).
Wong WW, Butte NF, Smith EO, Garza C & Klein PD (1989) Body composition of lactating women determined by anthropometry and deuterium dilution. Br J Nutr; 61: 25-33.
2. Halliday D & Miller DG (1977)
Precise measurement of total body water using tracer quantities of deuterium oxide. Biomed
Mass Spectrom; 4: 82-87.