Previous - Next
This is the old United Nations University website. Visit the new site at http://unu.edu
Moisture fluxes at land surfaces
Components of the Energy Budget
The nature of the land surface affects conditions in the lower layers of the atmosphere through its influence on the value of net radiation and through its effects on the transfer of momentum, moisture, and sensible heat between the air and the ground. These transfers are linked to one another and operate within the constraint of the energy balance at the surface. The energy budget equation can be written as
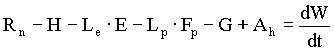(9)
in which energy fluxes from the atmosphere to the land surface are taken as positive. Rn, is the net radiative flux density at the particular surface (water, soil, snow, or vegetal canopy); H is the specific flux of sensible heat into the atmosphere; Le is the latent heat of vaporization and E the rate of evaporation; Lp is the thermal conversion factor for the fixation of carbon dioxide and Fp is the specific flux of carbon dioxide; C is the specific energy flux into the land surface; Ah is the energy advection into the surface layer and W is the energy stored in the surface layer.
On the space and time scales of general circulation models (100-500 km and 10-15 days), four of the seven terms in equation (9) can be assumed to be negligible compared to the remaining three terms. The advection term Ah may be significant in the case of rainfall on a snow surface or snowfall on a warm lake but is likely to be negligible otherwise for any area of appreciable extent. Table I shows representative values of the other terms of equation (9) for vegetation of about I m height under cloudless summer conditions in middle latitudes (Thom 1975).
TABLE 1. Typical energy budget over vegetation (Wm-2)
| Time |  |
 |
  |
G |  |
| Near sunrise | 0 | -8 | + 3 | - 5 | + 10 |
| Noon | 500 | 461 | + 12 | +25 | + 2 |
| Near sunset | 0 | +3 | +2 | +5 | - 10 |
| Midnight | - 50 | - 20 | - 3 | - 25 | - 2 |
If we average over a day to eliminate the diurnal variation, then the daily contribution of the energy flux into the canopy and of the rate of change of energy storage in the canopy and in the soil becomes negligible, so that the daily energy balance can be written
(10)
The term arising from carbon dioxide fixation may sometimes be of the order of 5% of global radiation but is more usually less than 1%. In practice this photosynthetic term is neglected except in direct studies of carbon dioxide exchange. The energy budget of equation (9) therefore reduces for the space and time scales of interest in GCM modelling to
(11)
The ratio of sensible heat flux to the vertical flux of latent heat (the Bowen ratio) is used to characterize the partition of the available energy between heat and moisture transfer to the atmosphere. Thus
(12)
where B is the Bowen ratio.
The net radiation available at the land surface depends on the nature of that surface. The net radiation is given by
(13)
where the first and second terms on the right-hand side of the equation represent available short-wave and long-wave radiation respectively. Rs is global short-wave radiation downwards on the land surface; a is the surface albedo or ratio of reflected to incident short-wave radiation; e is the long-wave emissivity of the surface; R. is the downward long-wave radiation; To is the surface temperature and sigma the StefanBoltzmann constant. The value of the albedo is strongly influenced by the nature of the surface cover: a varies from 0.1 for tropical rain forest to 0.8 for snow at high latitudes. In contrast, the emissivity does not vary widely, being between 0.9 and 1.0 for most natural surfaces.
Components of the Water Budget
Water balances can be attempted on a variety of space scales and a variety of time scales. In the coupling of general circulation models with land surface processes, the values for any individual grid square of rainfall and snowmelt and potential evaporation may be considered as given. Hydrologic modelling is required to provide the corresponding values of infiltration and percolation, surface and subsurface runoff, changes in soil moisture storage, and actual evapotranspiration. For the time scales of interest in climate modelling, changes in surface storage for snow-free areas are negligible compared with subsurface storage.
For any defined area we can then write
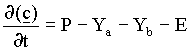(14)
where 
is a vector representing the state of the
soil moisture in various layers of the soil profile both
saturated and unsaturated; P(t) is the rate of precipitation;
E(t) is the rate of evaporation from water, soil, and vegetation;
Ya(t) is the direct storm runoff, which is too rapid
to be available for evaporation; and Yb(t) is the
subsequent baseflow, which is affected by evaporation. In any
part of the area in which the rate of precipitation P(t) is less
than the rate of potential infiltration Fp(t) at the
surface (or the rate of potential percolation of near-surface
soil layer), the direct runoff is assumed to be zero so that we
have
(15a)
and
(15b)
where F(t) is the rate of actual infiltration (or percolation). If, on the contrary, the rate of precipitation is greater than the rate of potential infiltration Fp(t) then the local rate of direct runoff will be given by
(16a)
and the rate of actual infiltration by
(16b)
In either of the two cases
(17)
which represents the assumption of negligible change in surface storage, and also
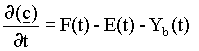(18)
which is the equation for soil moisture accounting.
The parametrization of potential infiltration starts from the physical equation for vertical flow in an unsaturated soil. For this situation, equation (8) above becomes
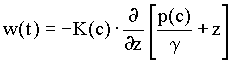(19)
where w(t) is the vertical velocity of unsaturated flow, K(c) is the unsaturated hydraulic conductivity, p(c) is the soil moisture pressure (which is negative in the unsatu rated zone), gama is the weight density of water, and z is the elevation. When combined with the continuity equation
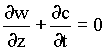(20)
equation (19) gives
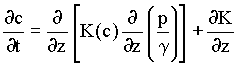(21)
which is known as Richards equation.
For dry soils the second term on the right-hand side of equation (21) is much smaller than the other two terms during the initial period of high rate infiltration. If this term is neglected, it can be shown that for surface ponding of a semi-infinite soil column the cumulative infiltration is given by
(22)
where A is a parameter (often referred to as the sorptivity) that depends on the initial soil moisture content and on the soil properties and B is a parameter that depends on the hydraulic conductivity. If the second term on the right-hand side of equation (21) is not neglected, a solution can be found by successive perturbations (Philip 1957, 1969). Brutsaert (1977) showed that a good approximation to the series solution is given by
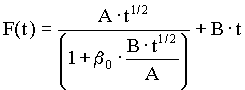(23)
where b0 is a parameter that depends on the pore-size distribution and that is of the order of b 0 = 2/3 for most field soils.
One could attempt to derive the values of A and B either by parametrization from the microscale of physical hydrology or by calibration on the basis of catchment records on as large a scale as possible. On the scale appropriate to general circulation modelling the effect of the approximations in equation (22) or equation (23) are negligible in relation to the uncertainty of the spatial variability in the physical parameters at the microscale. A possible approach at the catchment scale might be to use the fact that simplified solutions of equation (21) for an initial moisture content c0 uniformly distributed in a semi-infinite column indicate that sorptivity is proportional to (csat - c0) for a number of varying soil moisture relationships. Accordingly, one might attempt to examine whether at the large scale the derived sorptivity (A) was approximately proportional to the field moisture deficit and whether the constant of proportionality (B) could be related to major soil types.
Potential and Actual Evaporation
The problem of estimating actual evaporation when the moisture flux is soil controlled rather than atmosphere controlled remains a central problem in catchment hydrology. The combination approach pioneered by Penman (1948, 1963) represents a useful means of estimating evaporation independently rather than treating it as a residual in a waterbalance equation. In its original form the Penman estimate for potential evaporation is
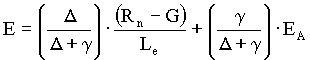(24)
where the first term on the right-hand side represents the equilibrium rate of evaporation very far downstream from the leading edge of a wet surface and EA represents the drying power of the air, which decreases downstream of the leading edge. The drying power EA is a function of the wind speed and the vapour pressure deficit at the air temperature.
Even when vegetation is well supplied with water the vegetal surface will not be wet except after precipitation. The rate of evaporation can be related to the gradient of specific humidity between the saturated air in the sub-stomata! cavities and the atmosphere above the canopy by
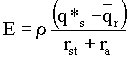(25)
where rst is the bulk stomata! resistance to the transfer of vapour from inside the stomata to the leaf surface and ra the aerodynamic resistance to the movement of vapour through the canopy air. Using the relationship between specific humidity (q) and vapour pressure (e)
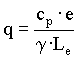(26)
we can derive the Penman-Monteith equation for a vegetated surface
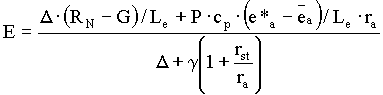(27)
which for rst = 0 is equivalent in form to equation (24).
It remains to consider the key question of the reduction of potential to actual evapotranspiration for the large space scales and long time scales appropriate to climate modelling. Long-term average relationships have been suggested on the basis of observed records, conceptual modelling at catchment scale, and parametrization of the equations of physical hydrology derived for conditions at a point. The empirical approach can be illustrated by the three examples of the methods proposed by Turc, by Pike, and by Budyko for estimating the annual evaporation from the annual potential evaporation and the annual precipitation.
Turc (1954, 1955) assumed that there would be a limiting rate of evaporation as annual precipitation increased and, on the basis of records of 250 catchments in different climatic regimes, proposed the formula
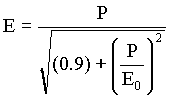(28)
where E, E0, and P are, respectively, the annual values of actual evapotranspiration, maximum possible evapotranspiration (based on a cubic relationship with mean annual temperature), and precipitation. Pike (1964) replaced the estimate of limiting evaporation by the Penman estimate of open-water evaporation and found that replacing 0.9 by 1.0 gave better results for Malawi.
Budyko (1948, 1971) found that data from the water balance of a number of catchments were intermediate between the exponential relationship proposed by Schreiber in 1904 and the hyperbolic tangent relationship proposed by Ol'dekop in 1911. Accordingly, he proposed the geometric mean of the two relationships. Thus
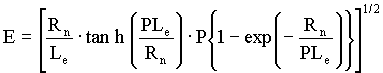(29)
This relationship was checked first for 29 European rivers (Budyko 1951) and then for 1,200 regions for which precipitation and runoff data were available (Budyko and Zubenok 1961).
The Turc and Pike equations can be directly compared with the Budyko equation by assuming that for moist conditions over a large area
(30)
and writing all three equations in the form of E/E0, as a function of P/E0 or P/Rn, which is the reciprocal of the Budyko index of dryness. This enables us to compare all three equations on figure 4, where the evaporation efficiency is plotted against the ratio of precipitation to maximum possible evaporation. The characterization of the biomes (desert, steppe, forest, tundra) in figure 4 by the value of P/E0 (= LeP/Rn) is due to Budyko (1971).
Conceptual catchment models formulated at a catchment scale are essentially methods of water storage accounting and a key element in their operation is soil moisture accounting. The simplest form of soil moisture accounting is a single storage element and the simplest relationship between actual evaporation and soil moisture storage is one that is linear below a threshold value (Thornthwaite and Mather 1955; Budyko 1955, 1956). Hydrological catchment models use multi-layer soil moisture accounting and allow for varying values for potential infiltration and potential evaporation in the catchment area (Fleming 1975). Conceptual models have also been developed to represent the soil-plant-atmosphere system (Halldin 1979; Fritschen 1982), but a review of these approaches is outside the scope of the present paper.
The main attempt to parametrize from physical hydrology to catchment scale and to GCM scale has been made by Eagleson (Eagleson 1978; Milly and Eagleson 1982a, 1982b; Andreou and Eagleson 1982). This research work is reviewed briefly under "Macro-Hydrology" below. In another approach to macroscale formulation, Bouchet (1963) considered regional evaporation under conditions of a fixed energy budget and suggested that the actual evaporation and the apparent potential evaporation based on actual atmospheric conditions would be complementary to one another. Morton (1965, 1975) and Brutsaert and Stricker (1979) have applied this concept to the estimation of regional evapotranspiration.
