Figure 13 illustrates what is understood by the terms shape and size in this context. Size refers to the dimensions and masses of the body. Shape is used to refer to the relative skeletal dimensions of the body and in this mode it is commonly expressed as a ratio of two linear dimensions. Relativity suggests that differences due to size are excluded but this is rarely the case with such simple ratio scaling. In morphology and morphometrics shape is regarded as three-dimensional, unaltered by scale, transformation or rotation, and bivariate comparisons are considered as proportions or proportionality. The three attributes of size, proportionality and shape make up the body frame, separate from the characteristic of composition. Soft tissues do contribute to shape, and particularly to the differences between the sexes, but for these purposes shape has been separated from composition.
The most common bivariate index of shape is the Cormic index, sitting height. total height (SH/S). It is a measure of the relative length of the trunks or legs and varies between individuals and groups. For a 70 kg, 1.75 m individual, BMI = 22.9 kg/m2, if sitting height is held constant and leg length varied to produce a range of ratios from 0.48 to 0.55 found within and between populations, the relationship between BMI and SH/S is as shown in Fig. 14. This demonstrates that variations in SH/S found in or between different population groups may be associated with variations in BMI of some 5kg/m2, with weight and composition being kept constant. The same degree of variation exists for the representative women considered earlier. The mean SH/S for European and Indo-Mediterranean populations is about 0.52. Africans have proportionally longer legs, in general, with ratios around 0.51. Asian and Far Eastern populations have proportionally shorter legs and means of 0.53-0.54 (Pheasant, 1986). However, there is considerable variation within populations and within these major groupings.

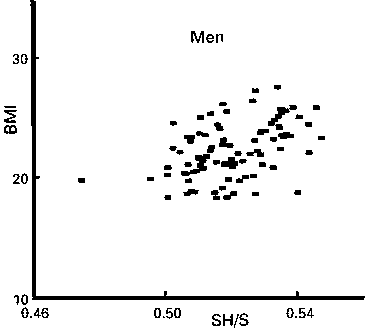
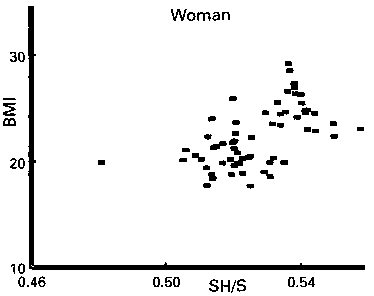
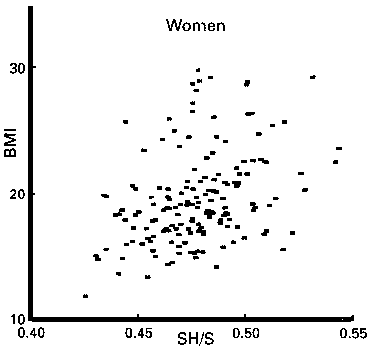
To determine if this pattern and related differences in BMI on SH/S obtain in groups and individuals data were collected from the literature on mean body weight, height and sitting height, and where available, SH/S. Data on 95 samples of men and 63 samples of women representing 18 000 individuals were taken from 46 papers. Scatter plots are shown in Fig. 15. The correlation coefficients are 0.45 for men (P < 0.001) and 0.56 for women (P < 0.001). Analysis of covariance showed no significant difference in regression coefficient (F = 2.3, d.f. 1, 153, n.s.) or intercept (F = 2.4, d.f. 1, 154, n.s.) in the sexes. Linear regression analysis on the combined data indicates a difference of BMI of 0.90kg/m2 for a 0.01 difference in SH/S. The model predicted a change of 0.88kg/m2 for a 0.01 difference in SH/S.
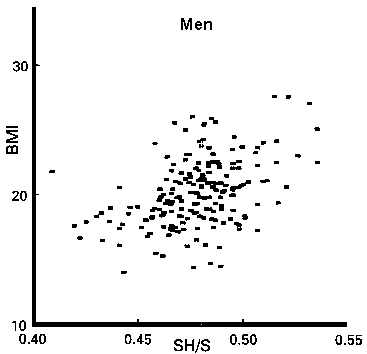
Population group differences exist along the lines indicated above and elsewhere (Pheasant 1986; Norgan, 1994 a,b). The outliers at the bottom of the range for SH/S are a group of Australian Aborigines (Abbie, 1967). These had extremely long legs. Data on individual Aborigines made available by the Australian Institute for Aboriginal and Torres Straits Islander Studies, Canberra, shown in Fig. 16 and described in detail elsewhere (Norgan, 1994 a,b), were significantly correlated, r = 0.43, for the 187 men (P < 0.001), although less well than the mixed group data for the 162 women, r = 0.37 (P < 0.001).
Lower correlations were also found in the individual data of 420 coastal Papua New Guineans (Harvey, 1974), r = 0.19 in men, 0.31 in women. Entering SH/S into a multiple regression of BMI on % fat and age increased the proportion of the variance explained by <2%. In these Papua New Guineans, biacromial diameter (shoulder width) was more highly correlated with BMI than SH/S in men, r = 0.29 and women, r = 0.41. Grimley Evans & Prior (1969) found biacromial diameter to contribute significantly to the regression of BMI on skinfold thicknesses in two Polynesian populations. Small differences in biacromial diameter at a given stature would, it was calculated, have important effects on the mean BMI of a group. This led the authors to conclude that comparisons of weight:height indices between ethnic groups may be misleading. However, the regression coefficients did not differ between the Polynesians and New Zealanders. Correlations between BMI and SH/S of up to 0.26 in adolescents and of 0.21 in men have been found from the data of the National Health and Nutrition Examination Survey 1 of 14 892 individuals in the USA (Garn, Leonard & Rosenberg, 1986).
Rural and low socio-economic status Indians have some of the lowest mean BMI and greatest proportions of the population with BMI below 16kg/m2. However, their SH/S are similar to those in European groups. Shape is unlikely to be playing a role here although Indian populations are very diverse.
More work on the contribution of shape to the BMI above and beyond those of size and composition is required to determine its importance before it can be set aside. However, what the Aborigine data show is that even within a quite distinct population group there are considerable inter-individual differences and ascribing a single value to a group to adjust or allow for the effects of shape appears unwarranted. Rather the values and variability in the data need to be established and taken into account in interpreting BMI.
In conclusion, low BMI approximates to low weight, FM and the functionally important FFM. There are significant differences in the relationships of body composition to BMI but for many purposes and over the range of BMI 20-25 kg/m2 these may not be important. Shape, as described by SH/S ratios, affects the BMI and its interpretation. To interpret BMI in terms of body composition more specifically it is necessary to take into account sex, age and ethnicity.
Abbie AA (1967): Skinfold thicknesses in Australian Aborigines. Archeol. Phys. Anthropol. Oceania 3, 223-231.
Allen TH, Peng MT, Chen UP, Huang TF, Chang C & Fang HS (1956): Prediction of blood volume and adiposity in man from body weight and cube of height. Metabolism 5, 328-345.
Bindon JR & Baker PT (1985): Modernization, migration and obesity among Samoan adults. Ann. Hum. Biol. 12, 61-76.
Davies CTM, Barnes C, Fox RH, Ojikutu RO & Samueloff AS (1972): Ethnic differences in physical working capacity. J. Appl. Physiol 33, 726-732.
Day J. Bailey A & Robinson R (1979): Biological variations associated with change in life style among the pastoralist and nomadic tribes of East Africa. Ann. Hum. Biol 6, 29-39.
Deurenberg P. Westrate JA & Seidell JC (1991): Body mass index as a measure of body fatness: age-specific prediction formulas. Br J. Nutr 65, 105-114.
Durnin JVGA & Womersley J (1974): Body fat assessed from the total body density and its estimation from skinfold thickness: measurements on 481 men and women aged from 16 to 72 years. Br. J. Nutr. 32, 77-97.
Elsner RW (1963): Skinfold thicknesses in primitive peoples native to cold climates. Ann. NY Acad. Sci. 110, 503-514.
Forbes GB (1987): Lean body mass - body fat interrelationships in humans. Nutr. Rev. 45, 225-231.
Gam SM, Leonard WR & Rosenberg K (1986): Body build dependence, stature dependence and influence of lean tissue on the body mass index. Ecol. Food Nutr 19, 163-165.
Garrow JS & Webster J (1985): Quetelet's index (W/H2) as a measure of fatness. Int. J. Obesity 9, 299-306.
Grimley Evans J & Prior IAM (1969): Indices of obesity derived from height and weight in two Polynesian populations. Br J. Prev. Soc. Med. 23, 56-59.
Harvey RG (1974): An anthropometric survey of growth and physique of the populations of Kar Kar Island and Lufa subdistrict, New Guinea. Phil. Trans. R. Soc. B268, 279-292.
Hiernaux J & Boedhi Hartono D (1980): Physical measurements of the adult Hadza of Tanzania. Ann. Hum. Biol. 7, 339-346.
Imminck MDC, Flores A & Diaz EO (1992): Body mass index, body composition and the chronic energy deficiency classification of rural populations in Guatemala. Eur. J. Clin. Nutr. 46, 419-427.
Keys A, Fidanza F. Karvonen MJ, Kimura N & Taylor HL (1972): Indices of relative weight and obesity. J. Chron. Dis. 25, 329-343.
Micozzi MS (1990): Applications of anthropometry to epidemiologic studies of nutrition and cancer. Am. J. Hum. Biol. 2, 727-739.
Micozzi MS & Harris TM (1990): Age variations in the relation of body mass indices to estimates of body fat and muscle mass. Am. J. Phys. Anthropol 81, 375-379.
Micozzi MS, Albanes D, Jones DY & Chumlea WC (1986): Correlations of body mass indices with weight, stature, and body composition in men and women in NHANES I and II. Am. J. Clin. Nutr. 44, 725-731.
Norgan NG (1990): Body mass index and energy stores in developing countries. Eur J. Clin. Nutr. 44 (Suppl. 1), 79-84.
Norgan NG (1991): Anthropometric assessment of body fat and fatness. In Anthropometric assessment of nutritional status, ed. J. Himes, pp.197-212. Alan R. Liss, New York.
Norgan NG (1994a): Interpretation of low body mass indices in Australian Aborigines. Am. J. Phys. Anthropol. 94, 229-237.
Norgan NG (1994b): Relative sitting height and the body mass index. Ann. Hum. Biol. 21, 78-82.
Norgan NG & Ferro-Luzzi A (1982): Weight-height indices as estimators of fatness in men. Hum. Nutr: Clin. Nutr 36C, 63-72.
Pheasant S (1986): Bodyspace: anthropometry, ergonomics and design. London: Taylor & Francis.
Prior JAM, Hooper A., Huntsman JW, Stanhope JM, Salmond CE (1977): The Tokelau Island migrant study. In Population structure and human variation, ed. GA Harrison, pp. 165-186. Cambridge: Cambridge University Press.
Revicki DA & Israel RG (1986): Relationship between body mass indices and measures of body adiposity. Am. J. Publ. Hlth 76, 992-994.
Roche AF, Siervogel RM, Chumlea WC & Webb P (1981): Grading body fatness from limited anthropometric data. Am. J. Clin. Nutr 34, 2831-2838.
Sanjeev, Indech GD, Indar Jit & Johnston SE (1991): Skinfold thicknesses, body circumferences and their relationship to age, sex, and socioeconomic status in adults from Northwest India. Am. J. Hum. Biol 3, 469-477.
Siri WE (1961): Body composition from fluid spaces and densitometry. In Techniques for measuring body composition, eds J Brozek & A Henschel, pp. 223-244. Washington DC: National Academy of Sciences.
Smalley KJ, Knerr AN, Kendrick ZV, Colliver JA & Owen OE (1990): Reassessment of body mass indices. Am. J. Clin. Nutr. 52, 405-408.
Snedecor GW & Cochrane WG (1967): Statistical methods, 6th edn. Ames: Iowa State University Press.
Valencia ME, Moya SY, McNeil G & Haggarty P (1992): Basal metabolic rate and body composition in Mexican males of different body mass index. Proc. Nutr Soc. 51, 69 (Abstract).
Womersley J & Durnin JVGA (1977): A comparison of the skinfold method with the extent of 'overweight' and venous weight-height relationships in the assessment of obesity. Br. J. Nutr 38, 271-284.
Scrimshaw: I wonder why no attention is given to the analyses of creatinine height index. Viteri, in Guatemala, observed that the 24h creatinine excretion in well-nourished children was 90-100% of controls of the same height. Those with kwashiorkor had an index of <70%, those with marasmus were even lower and those who had had measles in the preceding weeks had creatinine excretion in the range of 70-90% - a clear indication of loss of lean body mass. I know there are not many measurements in the literature, but with such a direct relationship should we not encourage the collection of that type of information, which in some populations should not be difficult to collect?
Waterlow: I agree. The creatinine index can give an index of the different compartments of lean body mass. There are practical problems in collecting 24h urines. My own experience is that the importance of a meat-free diet is grossly exaggerated. In our studies on an expedition into the high Andes, there was a huge variation in daily intake but the within-subject variation in creatinine excretion was <5%.
Norgan: We must be careful to distinguish between children and adults in the creatinine/height correlation. The MUAC is not particularly easy to measure because you need to identify the arm landmarks and the midpoint very accurately. Perhaps calf circumference would be easier because the widest point is easy to identify.
Waterlow: Isn't calf circumference also a measure of physical activity? And does it have less fat than the arm?
Norgan: It is difficult to increase calf circumference by training exercises. The skinfolds of the calf are very tight and difficult to measure. John Cotes in the English IBP contribution used radiographs with thigh circumference as an index of muscularity.
Shetty: It is difficult to assume that everybody below a BMI of 18.5 is chronically energy deficient (CED). It is likely that this cut-off point could be strengthened with an additional anthropometric index. The MUAC may be a useful additional index.
Branca: I would like to suggest a further use of MUAC. If we are using BMI to show the difference between the energy stores in different populations then a more useful indicator of muscle mass is arm muscle area which can exclude the fat. It needs two extra measurements, the MUAC and the triceps skinfold. There are data available in tabular form from NHANES II and III and this would allow standardization from different populations which could be expressed as Z scores of arm muscle area.
Berdasco: We show elsewhere correlations between BMI and different areas, and with upper arm fat area we get the best correlation.
Mascie-Taylor: I would be happier with the meta-analyses that Norgan has presented if they had been weighted for sample sixe and with the age effects dealt with separately. There is also some evidence of curvilinearity in the relationship between BMI and the percentage fat.
Norgan: It is a good idea to deal with the weighting and I can do that, but to deal with the age would mean analysing groups only one-quarter of the present size.
Ferro-Luzzi: We are assuming that BMI is independent of height but if we go back to Key's original paper on the Quetelet Index there was a long list of populations where BMI was independent of height but in other populations in developing countries BMI is not independent of height. Height may be affected by undernutrition and this could explain some of the heterogeneity between populations. We are still looking for an index which is simple but not crude.
James: I visualize two individuals, one is square and big boned and one is not, but they both have the same BMI between 17 and 18.5. However, we would consider them to have CED if their MUAC was also below a value 'x'. There would then be two requirements to categorize CED. I would then think you only need to measure MUAC because that is the plastic variable that you are truly interested in.
Naidu: It is obvious that there are two distributions of adults with young adults under 35 in one group and the other group, mainly over 35, who are less active. I have data on large numbers and there is no indication that MUAC will lead us anywhere because MUAC ranges from 23.2 to 24 and BMI ranges from 18.5 to 19.6. Do we place any trust in the 1 cm difference in MUAC? - the variability is very high and the sensitivity is no better than BMI.
Scrimshaw: You will remember from previous meetings that as the availability of the diet energy goes down, activity decreases before the BMI falls, so comparisons of activity levels of populations in developing and industrialized countries (which are not under dietary constraint) are not applicable to the interpretation of a low BMI.