Contents
-  Previous - Next
Previous - Next
This is the old United Nations University website. Visit the new site at http://unu.edu
Appendix: Calculation of linear and quadratic
contrasts
The following formulae were used
to calculate the contrasts (L) and their variance (V) and
confidence intervals (Cl) [22] used in estimating secular trends
in the length of the three-year-old Guatemalan children studied:
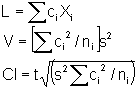
where
c = coefficients (see below)
X = mean length at three years of age
n = sample size
i = time of measurement (= 7)
s² = estimated variance
The coefficients were obtained by
the method proposed by Robson [15] for the construction of
polynomials for unequally spaced data (table A1).
TABLE A1. Coefficients for linear
and quadratic contrasts
| |
Spacing |
Coefficients |
| Linear |
Quadratic |
| 1968 |
0 |
-0.43 |
+0.56 |
| 1969-70 |
2 |
-0.31 |
+0.20 |
| 1971-72 |
4 |
-0.19 |
-0.07 |
| 1973-74 |
6 |
-0.06 |
-0.28 |
| 1975-76 |
8 |
+0.06 |
-0.41 |
| 1977 |
9 |
+0.12 |
-0.44 |
| 1988 |
20 |
+0.81 |
+0.44 |
As an example of the use of the
methodology, we show the calculation of the linear contrast and
its confidence interval for the atole group. Table A2 gives the
calculation of the summations 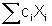 and
and 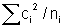 . From this:
. From this:
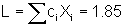
Then, using s² = 19.78,
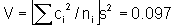
and
Cl = 0.61
Thus, the contrast = 1.85 ± 0.61
(1.24, 2.46).
TABLE A2. Calculation of 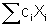 and
and 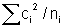
| |
ciXi |
ci2/ni |
| 1968 |
85.14
x -0.435801 |
-0.43582/196 |
| 1969-70 |
85.53
x -0.311286 |
-0.31132/82 |
| 1971-72 |
86.58
x -0.186772 |
-0.18682/100 |
| 1973-74 |
86.09
x -0.062257 |
-0.06222/117 |
| 1975-76 |
86.86
x +0.062257 |
0.06222/117 |
| 1977 |
86.55
x +0.124515 |
0.12452/35 |
| 1988-89 |
87.62
x +0.809345 |
0.80932/344 |
 |
1.85 |
0.0049 |
Acknowledgements
Data collection and analyses were
supported by NIH grant HD22440. The study was a collaborative
effort involving investigators at several institutions: R.
Martorell (principal investigator, originally at Stanford
University, now at Cornell University), J. Rivera (INCAP,
Guatemala), E. Pollitt (University of California at Davis), and
J. Haas (Cornell University).
References
- Tanner JM. The secular trend
towards earlier physical maturation. T Soc Geneesk
1966;44:524-39.
- Van Wieringen JC. Secular
growth changes. In: Falkner F. Tanner JM, eds. Human
growth: a comprehensive treatise. 2nd ed. Vol 3.
Postnatal growth. New York: Plenum Press, 1978:307-31.
- Himes JH, Mueller WH. Aging
and secular change in adult stature in rural Colombia. Am
J Phys Anthro 1917;46:275-79.
- Himes JH, Malina RM. Age and
secular factors in the stature of adult Zapotec males. Am
J Phys Anthro 1975;43:367-69.
- Malina RM, Buschang PH,
Aronson WL, Selby HA. Aging in selected anthropometric
dimensions in a rural Zapotec-speaking community in the
valley of Oaxaca, Mexico. Soc Sci Med 1982;16:217-22.
- Eveleth PB, Salzano FM, de
Lima PE. Child growth and adult physique in Brazilian
Xingu Indians. Am J Phys Anthro 1974;41:95-102.
- Miall WE, Ashcroft MT,
Lovell HG, Moore F. A longitudinal study of the decline
of adult height with age in two Welsh communities. Hum
Biol 1967;39:445-54.
- Cline MG, Meredith KE, Boyer
JT, Burrows B. Decline of height with age in adults in a
general population sample: estimating maximum height and
distinguishing birth cohort effects from actual loss of
stature with aging. Hum Biol 1989;61:415-25.
- Chumlea WMC, Garry PJ, Hunt
WC, Rhyne RL. Distributions of serial changes in stature
and weight in a healthy elderly population. Hum Biol
1988;60:917-25.
- Hertzog KP, Garn SM, Hempy
HO. Partitioning the effects of secular trend and aging
in adult stature. Am J Phys Anthro 1969;31:111-15.
- Klein RE, Habicht JP,
Yarbrough C. Some methodological problems in field
studies of nutrition and intelligence. In: Kallen DJ, ed.
Nutrition, development and social behavior: proceedings
of the conference on the assessment of tests of bebavior
from studies on nutrition in the western hemisphere.
Washington, DC: Government Printing Office, 1973:61-73.
- Rivera J. Martorell R.
Habicht JP, Ruel MT, Haas J. Long-term effects of
supplementary feeding in early childhood on body size and
composition. J Nutr (in press).
- Relethford JH, Lees FC. The
effects of aging and secular trend on adult stature in
rural western Ireland. Am J Phys Anthro 1981;55:81-88.
- Draper NR, Smith H. Applied
regression analysis. 2nd ed. Wiley Series in Probability
and Mathematical Statistics. New York: John Wiley, 1981.
- Robson D. A simple method
for constructing orthogonal polynomials when the
independent variable is unequally spaced. Biometrics
1959;15:187-91.
- Borkan GA, Hults DE, Glynn
RJ. Role of longitudinal change and secular trend in age
differences in male body dimensions. Hum Biol
1983;55:629-41.
- Himes JH, Mueller WH.
Age-associated statural loss and socioeconomic status. J
Am Geriatr Soc 1977;25: 171-74.
- Galloway A. Estimating
actual height in the older individual. J Forensic Sci
1988;33:126-36.
- Bergeron G. Social and
economic development in four Ladino communities of
eastern Guatemala: a comparative description. Food Nutr
Bull 1992;14(3):221-36.
- Hamill PVV, Drizd TA,
Johnson CL, Reed RB, Roche AF, Moore WM. Physical growth:
National Center for Health Statistics percentiles. Am J
Clin Nutr 1979; 32:607-97.
- World Health Organization.
Energy and protein requirements. Geneva: WHO, 1985.
- J. Wasserman W. Kutner MH.
Applied linear statistical models: regression, analysis
of variance, and experimental design. 2nd ed. Homewood,
Ill, USA: Irwin, 1985.
Contents
-  Previous - Next
Previous - Next
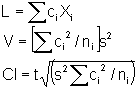
![]() and
and ![]() . From this:
. From this:![]()
![]()
![]() and
and ![]()